Directions (1-10): Find the wrong number in the following number series:
Q1. 3980, 3380, 2870, 2432, 2050, 1720, 1400
(a) 3380
(b) 3980
(c) 1400
(d) 1720
(e) 2050
Q2. 4, 6, 14, 51, 220, 1125, 6786
(a) 6
(b) 4
(c) 14
(d) 1125
(e) 6786
Q3. 268, 267, 275, 248, 312, 190, 403
(a) 268
(b) 267
(c) 190
(d) 403
(e) 248
Q4. 66, 32, 32, 48, 24, 60, 20
(a) 24
(b) 32
(c) 60
(d) 66
(e) 20
Q5. 325, 331, 343, 363, 393, 440, 491
(a) 491
(b) 393
(c) 331
(d) 325
(e) 440
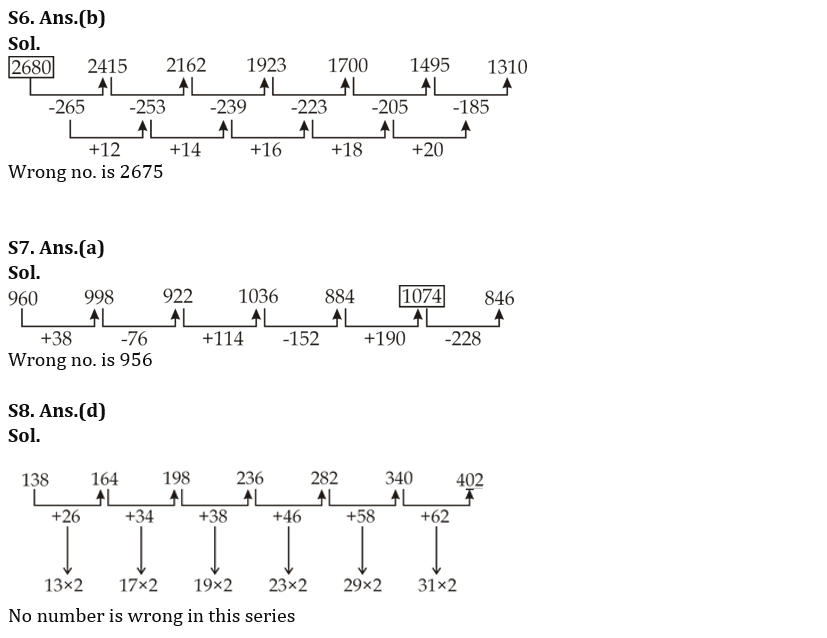
Q6. 2675, 2415, 2162, 1923, 1700, 1495, 1310
(a) None of the given number is wrong
(b) 2675
(c) 1495
(d) 1310
(e) 1923
Q7. 960, 998, 922, 1036, 884, 956, 846
(a) 956
(b) 884
(c) 922
(d) 998
(e) 960
Q8. 138, 164, 198, 236, 282, 340, 402
(a) 164
(b) 198
(c) 282
(d) None of the given number is wrong
(e) 402
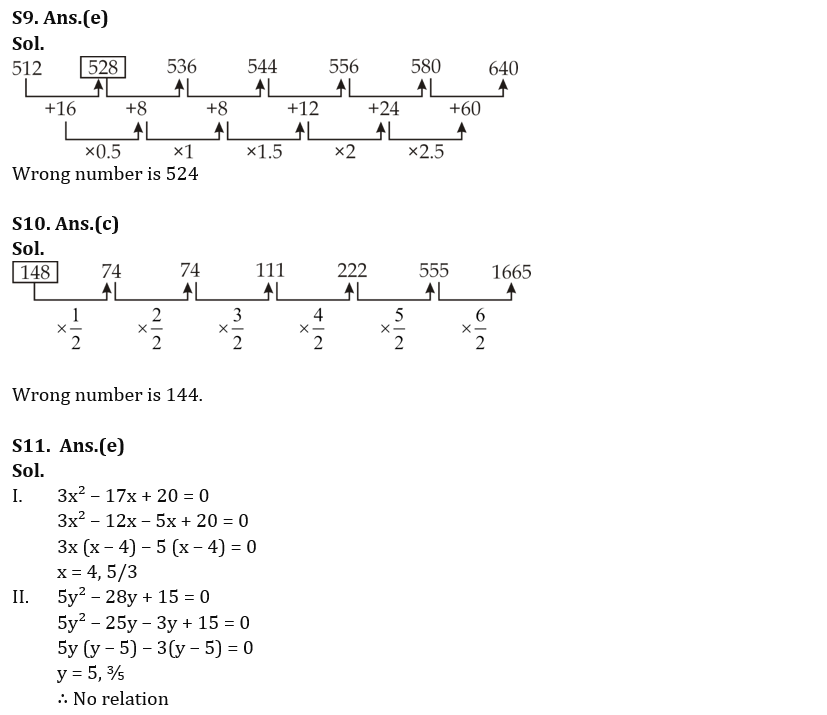
Q9. 512, 524, 536, 544, 556, 580, 640
(a) 512
(b) 536
(c) 556
(d) 640
(e) 524
Q10. 144, 74, 74, 111, 222, 555, 1665
(a) 1665
(b) 222
(c) 144
(d) 74
(e) None of the given number is wrong
Directions (11-15): In each of these questions, two equations (I) and (II) are given. You have to solve both the equations and give answer
Q11. (i) 3x² – 17x + 20 = 0
(ii) 5y² – 28y + 15 = 0
(a) if x > y
(b) if x ≥ y
(c) if x < y
(d) if x ≤ y
(e) if x = y or no relation can be established between x and y.
Q12. (i) 6x² = 11x – 4
(ii) 2y² = 15y + 8
(a) if x > y
(b) if x ≥ y
(c) if x < y
(d) if x ≤ y
(e) if x = y or no relation can be established between x and y.
Q13. (i) 2 (x – 3)² = (x – 3)
(ii) 5y² + 6y = –1
(a) if x > y
(b) if x ≥ y
(c) if x < y
(d) if x ≤ y
(e) if x = y or no relation can be established between x and y.
Q14. (i) 7x² + 61x + 40 = 0
(ii) 5y² – 13y – 28 = 0
(a) if x > y
(b) if x ≥ y
(c) if x < y
(d) if x ≤ y
(e) if x = y or no relation can be established between x and y.
Q15. (i) x² – 11x + 24 = 0
(ii) 2y² – 9y + 9 = 0
(a) if x > y
(b) if x ≥ y
(c) if x < y
(d) if x ≤ y
(e) if x = y or no relation can be established between x and y.
Solutions


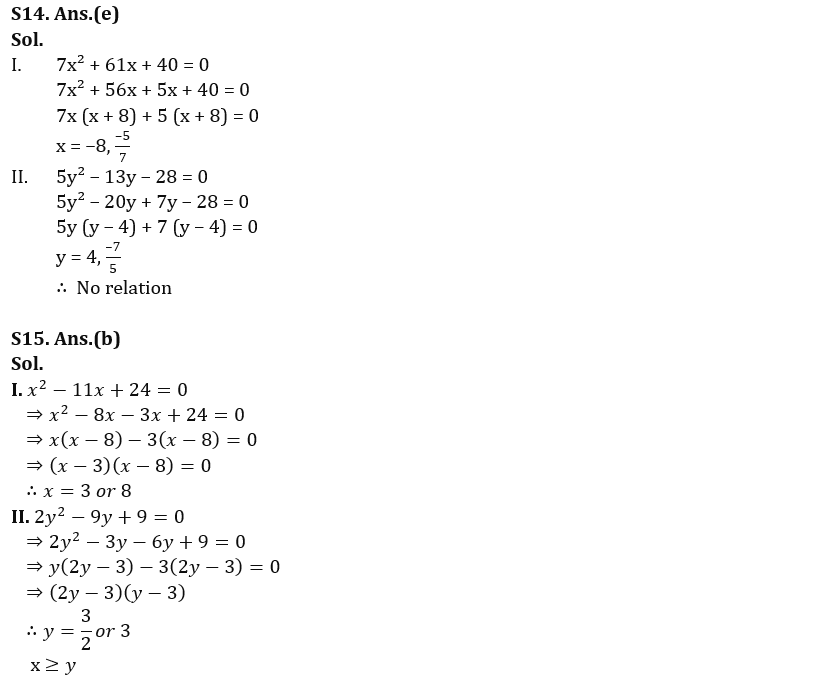





 Quantitative Aptitude Quiz For Bank Main...
Quantitative Aptitude Quiz For Bank Main...
 Quantitative Aptitude Quiz For Bank Foun...
Quantitative Aptitude Quiz For Bank Foun...




