Quantitative Aptitude Quiz For IBPS PO Mains 2022- 23nd October |

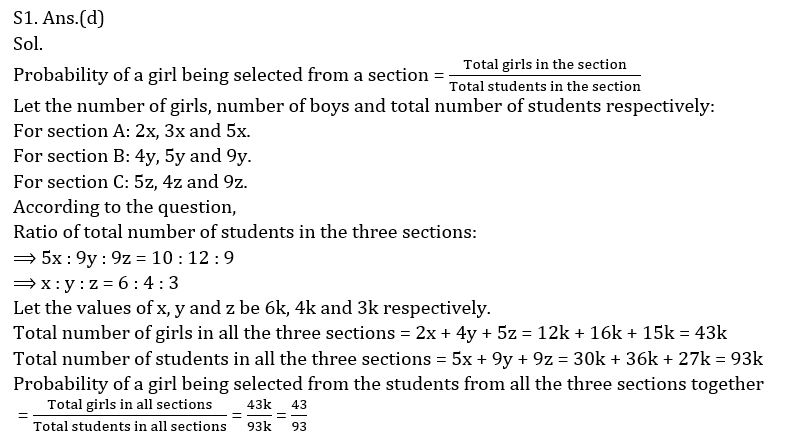
Directions (1-2): There are three sections A, B and C in a class. Every section has some boy and some girl students in it. Probability of a girl being selected when one student is selected randomly from section A is 2/5, that from section B is 4/9 and that from section C is 5/9.

Q1. If the ratio of total number of students in sections A, B and C is 10 : 12 : 9, then what is the probability of a girl being selected when one student is selected randomly from the students from all the three sections together?
(a) 14/31 (b) 11/23 (c) 13/31
(d) 43/93 (e) Cannot be determined

Q2. If the number of girls in sections A is same as the number of boys in section C, then what is the ratio of number of boys in section A to the number of boys in section C?
(a) 2 : 3 (b) 3 : 4 (c) 3 : 2
(d) 4 : 3 (e) None of these

Directions (3-5): Given below are two pie charts. Pie chart (1) shows the percentage distribution of milk in five vessels out of the total milk in these five vessels. Pie chart (2) shows the percentage distribution of water in same five vessels out of total quantity of water in these five vessels.

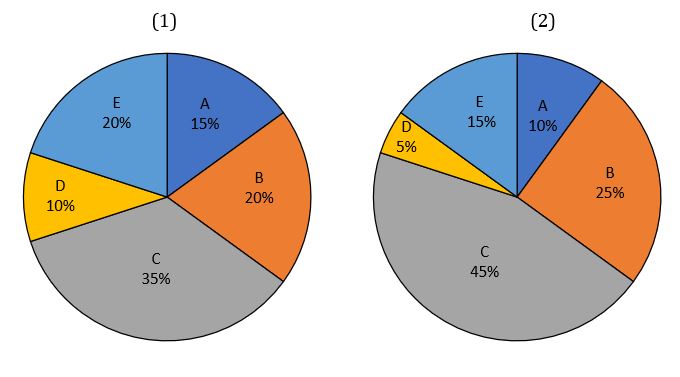
Note: Ratio of total milk to total water in these five containers is 2 : 1.

Q3. A shopkeeper pours the mixture of vessel A and B into another vessel F. Vessel F contains water only which is equal to 25% of water of vessel B. If shopkeeper professes to sell the whole mixture at the cost price of pure milk and cost price for shopkeeper is due to milk only, then find the percentage profit of shopkeeper in selling whole mixture.
(a) 58 (13/14)%
(b) 3 (13/15)%
(c) 54 (13/15)%
(d) 53 (13/14)%
(e) 55 (20/21)%

Q4. Mixture of vessel A and C are mixed into another vessel M. If 62 liters of the mixture M is taken out and replaced with 17 L of water, the ratio of milk to water in M becomes 6 : 5. Find the quantity of milk in vessel B.
(a) 60 L
(b) 20 L
(c) 40 L
(d) 45 L
(e) 50 L

Q5. All the contents of mixture from all vessels except C is poured into bigger vessel and from vessel C, only 115 liters of mixture is taken out and poured into bigger vessel, then ratio of milk and water in bigger vessel becomes 9 : 4. Find the total quantity of water in all five vessels.
(a) 550 L
(b) 500 L
(c) 600 L
(d) 650 L
(e) 700 L

Directions (6-10): The following questions are accompanied by three statements (A), (B), and (C). You have to determine which statement(s) is/are sufficient necessary to answer the questions

Q6. A bag contains some balls of red, black and blue colors. 3 balls are drawn randomly from it. What is the probability that the balls drawn are of three different colors?
A. Ratio of black and blue colored balls is 4 : 5.
B. Total number of red and black balls is 5 more than that of blue colored balls.
C. Total number of balls in that bag is 35 and probability of selecting blue colored balls is 3/7.
(a) A and either B or C
(b) Any two of them
(c) Only A and C together
(d) Question can’t be answered even after using all the information
(e) All statements are required

Q8. What is the value of a two digit number?
A. The sum of the number and its square is 25 times the number itself.
B. The number obtained after interchanging the digits is greater than the original number by 18.
C. The ratio of the value of the number and the sum of the digits of that number is 4 : 1.
(a) Either A alone or B and C together
(b) All the three together
(c) Any one of the three
(d) All the three together are not sufficient
(e) Either A or B alone

Q9. What is the selling price of an article if the marked price of that article is 60% above the cost price of that article?
A. The article is sold at 12% profit and there are two successive discounts of 20% and 12 (1/2)% on marked price.
B. Difference of marked price and the cost price of that article is Rs 480.
C. The marked price is Rs.384 more than the selling price of that article.
(a) Only B and C together
(b) Any two of three are sufficient
(c) Either A and B together or A and C together
(d) Only A and C together
(e) All the three together

Q10. Three friends A, B and C invested in a scheme. Find the profit share of A?
A. Investment of A, B and C is in the ratio 9: 12: 10 and share of C in profit is Rs.1800
B. A and B invested Rs.8100 and Rs.10800 for 10 months respectively and profit share of B is 100% of the profit share of C.
C. A invested Rs 900 more than that of C and investment period of B is 10 months.
(a) A and B together only
(b) Either A and B or A and C
(c) Any two of them
(d) Either B alone or A and C together
(e) Either A and C or B and C

Solutions