Quantitative Aptitude Quiz For Bank Mains Exam 2024-07th february

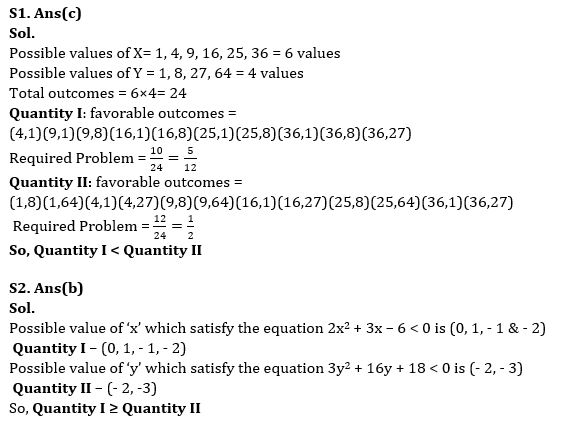
Q1. ‘X’ is a perfect square less than 40, and ‘Y’ is a perfect cube less than 100.
Quantity I – What will be probability of X > Y.
Quantity II – What will be probability that the sum of X & Y is an odd number.
(a) Quantity I > Quantity II
(b) Quantity I ≥ Quantity II
(c) Quantity II > Quantity I
(d) Quantity II ≥ Quantity I
(e) Quantity I = Quantity II or Relation cannot be established

Q2. If 2x² + 3x – 6 < 0 and 3y² + 16y + 18 < 0, and ‘x’ and ‘y’ are integers, then
Quantity I – Value of ‘x’
Quantity II – Value of ‘y’
(a) Quantity I > Quantity II
(b) Quantity I ≥ Quantity II
(c) Quantity II > Quantity I
(d) Quantity II ≥ Quantity I
(e) Quantity I = Quantity II or Relation cannot be established

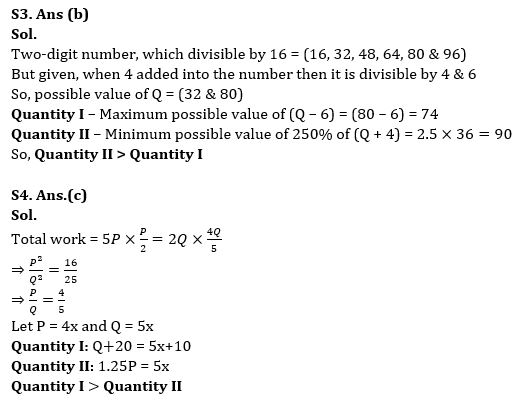
Q3. ‘Q’ is two-digit number divisible by 16, and when 4 added into the number then it is divisible by 4 & 6.
Quantity I – Maximum possible value of (Q – 6).
Quantity II – Minimum possible value of 250% of (Q + 4)
(a) Quantity I > Quantity II
(b) Quantity I < Quantity II
(c) Quantity I ≥ Quantity II
(d) Quantity I ≤ Quantity II
(e) Quantity I = Quantity II or No relation

Q4. ‘A’ type of 5P taps can fill a tank in P/2 hours, while ‘A’ type of 2Q taps can fill the same tank in 4Q/5 hours.
Quantity I: Value of ‘Q+20’.
Quantity II: Value of ‘1.25P’
(a) Quantity I ≥ Quantity II
(b) Quantity I = Quantity II or No relation
(c) Quantity I > Quantity II
(d) Quantity I < Quantity II
(e) Quantity I ≤ Quantity II

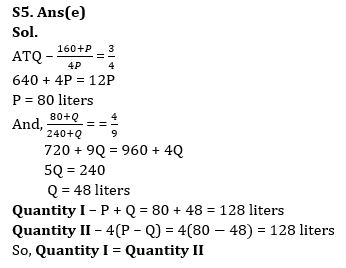
Q5. A container contains 160 lit of pure milk and P liters of water. Ratio of 25% of mixture to the initial quantity of water is 3 : 4 and when Q liters of water is added to it ratio of total mixture to water becomes 9 : 4.
Quantity I – Find the P + Q.
Quantity II – Find the 4(P – Q).
(a) Quantity I > Quantity II
(b) Quantity I ≥ Quantity II
(c) Quantity II > Quantity I
(d) Quantity II ≥ Quantity I
(e) Quantity I = Quantity II or Relation cannot be established

Solutions

                                                              . .               .